- ...P
![[*]](footnote.png)
- nagel@inf.ethz.ch.
ETH Zentrum IFW B27.1, 8092 Zürich, Switzerland
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...
![[*]](footnote.png)
- martin.shubik@yale.edu
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...
![[*]](footnote.png)
- paczuski@alf.nbi.dk
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...
![[*]](footnote.png)
- bak@alf.nbi.dk
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ....
![[*]](footnote.png)
- In this paper, we will also use
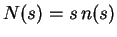 for the cluster
size distribution in logarithmic bins, in particular for the
figures.
for the cluster
size distribution in logarithmic bins, in particular for the
figures.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... size.
![[*]](footnote.png)
- An establishment is ``a single physical location at
which business is conducted. It is not necessarily identical with a
company or enterprise, which may consist of one establishment or
more.'' [12].
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... here.
![[*]](footnote.png)
- Remember again, that slopes from log-log plots in logarithmic bins
are different by one from the exponent in the distribution. So
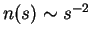 corresponds to a slope
corresponds to a slope 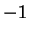 both in the
accumulated distribution
both in the
accumulated distribution 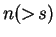 and when plotting logarithmic bins
and when plotting logarithmic bins
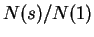 .
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ...fig:non-spatial-gpl).
![[*]](footnote.png)
- Note that the approach in this section corresponds to measuring the
cluster size distribution every time we give an agent back to the
system, while in the simulations we measured the cluster size
distribution only just before a cluster was picked for deletion. In
how far this is important is an open question; preliminary
simulation results indicate that it is important for the spatial
case with injection but not important for the non-spatial case in
this section.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... business.
![[*]](footnote.png)
- In this model no accumulation of assets is allowed. This
simplification will be relaxed in future work.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... shop.
![[*]](footnote.png)
- The simplification that customers react to price changes only is
useful because it leads to the separation of time scales between
consumer behavior and firm behavior.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... customer.
![[*]](footnote.png)
- If all prices in the system are more than
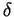 below one,
then the model is not well-defined. In the limit of large systems
and when starting with prices above one, such a state cannot be
reached via the dynamics. - Also note that if the model allowed
credit, the exit of such a company would be delayed, allowing losses
for limited periods of time.
below one,
then the model is not well-defined. In the limit of large systems
and when starting with prices above one, such a state cannot be
reached via the dynamics. - Also note that if the model allowed
credit, the exit of such a company would be delayed, allowing losses
for limited periods of time.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
- ... model.
![[*]](footnote.png)
- Furthermore, models such as the ones discussed in this paper often
have a so-called upper critical dimension, where some aspects of the
model become the same as in infinite dimensions. This upper critical
dimension often is rather low (below 10).
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)
![[*]](footnote.png)